In Mathematics, teachers can choose any appropriate methodology to develop students' ability to understand and use mathematics terminology.
The principles of any robust vocabulary instruction can be applied to the domain of mathematics (Pierce & Fontaine, 2009). The examples below demonstrate how two strategies might look in a Mathematics classroom. Other strategies to teach new terminology can be found in the other subject area sections of this Toolkit.
Joint construction of terminology
Joint construction (van Vondel et al., 2017) in mathematics can involve the teacher working collaboratively with students to develop meaning for required mathematical terminology. The teacher can provide carefully selected examples and non-examples to prompt students' discussion of concepts or skills to provide meaning for given terminology.
The strategy involves:
- the teacher introducing a new mathematical term in the context of existing knowledge
Example
The teacher introduces the term 'factorise' and asks students what they know about this term.
To scaffold students, they could be asked to expand brackets of the form a(x+b), for example, 5(x+4) = 5x + 20.
The teacher then explains to students that the reverse process of 'expanding' is called 'factorising'.
- pairs of students discuss what they know about the term and write a preliminary definition for the term (e.g. Students may write "Factorise means write the factors in brackets"). Students are encouraged to include examples (e.g. provide an expression and the factorised form) to support their definition.
- the teacher asks students to share their definitions, and records the definitions on the board. Definitions could also be shared using a digital collaborative tool
- the teacher engages the class in discussion to work towards a refined and shared definition
- students compare their initial definition with the class definition, as well as a dictionary or textbook.
The Frayer model
The
Frayer model is a grid with four sections that can be used to support student understanding of new terminology. Below is an example of a completed Frayer model for 'pronumeral', which could be used in a Year 7 class (VCMNA251).
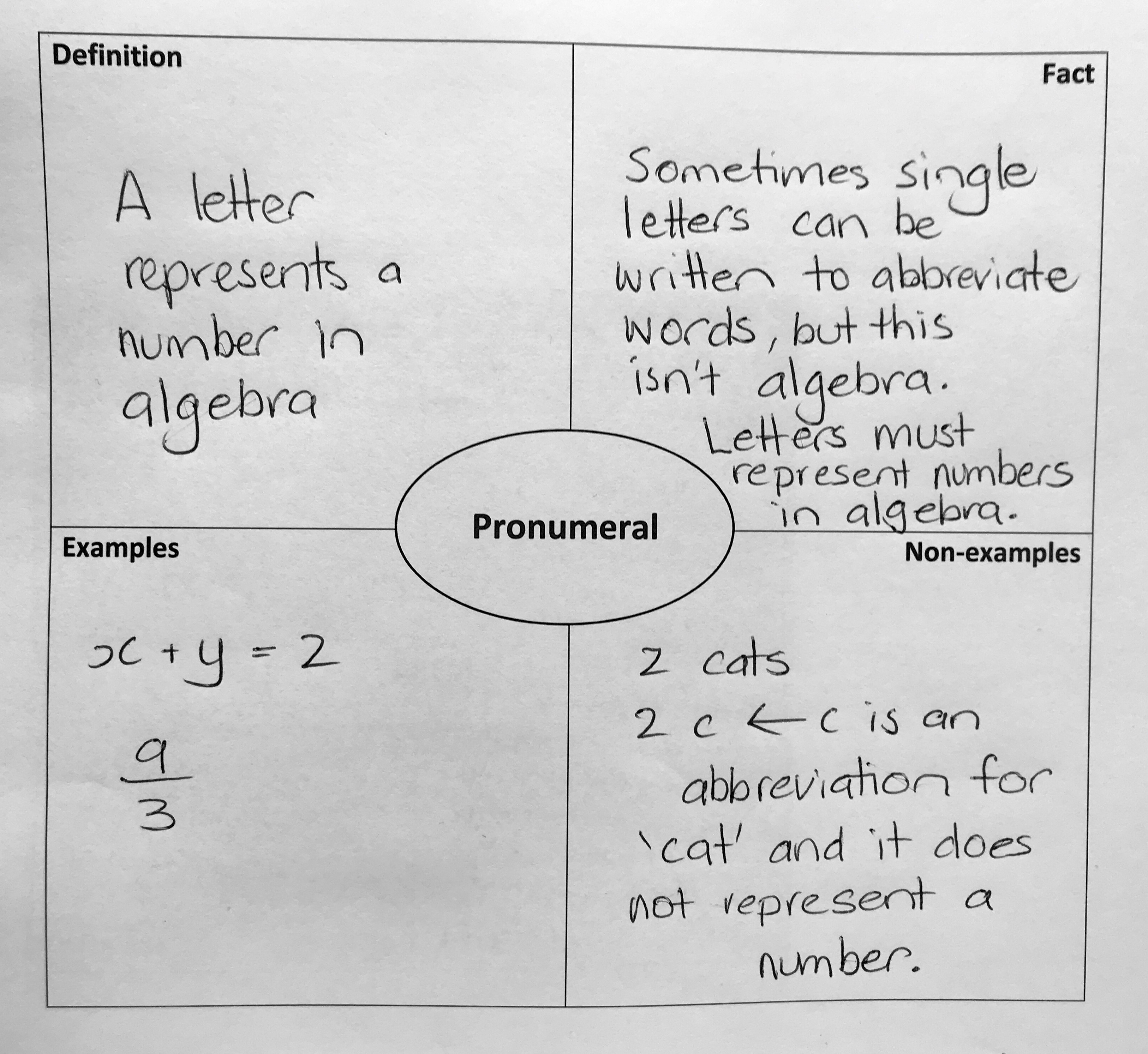 Frayer model being used to explore pronumerals
Frayer model being used to explore pronumerals
The Frayer model is especially helpful when supporting students to learn mathematical vocabulary that has other meanings in other subject areas, or when teaching new and difficult concepts (Rozzelle & Scearce, 2009).
The Frayer model can be adapted to suit various year levels and concepts.
Example using the Frayer model
For example, teachers could include a section that requires students to draw a symbolic representation or picture (Armstrong, Ming & Helf, 2018), as in the example below for a Year 9 class on trigonometry.
Sine, cosine and tangent ratios
Example
Book definition
| My definition
|
Draw a triangle with side lengths. Calculate the angles.
| Draw a triangle with one side length and angle. Calculate the other side lengths and angles.
|
Written description of where the triangles you drew might appear in the real world.
|