Students need to develop their ability to read and understand symbols used for operations. This helps students make sense of mathematical statements (i.e., expressions, equations) both numeric and algebraic.
For example, if two mathematical objects,
a and b, are to be added (or multiplied), then this can be written as a + b or b + a (for multiplication, a × b or b
× a), as both addition and multiplication are commutative. This differs to subtraction and division where the mathematical objects cannot be reordered, i.e. a - b cannot be read or rewritten as b - a, and a ÷ b cannot be read or rewritten as b ÷ a.
Understanding this strategy
One application of reading operations for meaning is in the performance of mental calculations.
Teachers should choose a calculation that is in the current topic and discuss the alternative ways of "reading the operation".
Teachers write calculation (numbers and operation) on board
Students discuss in pairs alternative ways of reading the calculation (both number notation and operations, as shown below in the example)
Class discussion of any terms relevant for the given calculation. For example,
- commutativity
- common denominator
- negative
- subtraction
Discuss how different ways of reading expressions can help with mental calculations.
Examples of reading operations for meaning
The tables below provide examples which can be used to prompt class discussion of reading operations for meaning.
Addition
Example
Example
| Common reading
| Alternative reading for meaning
|
|---|
|
3 + 199 | 3 plus 199
| As addition is commutative, start with199 add 3 more; result of 202.
|
|---|
3/8 +
1/4
| 3 over 8 plus 1 over 4
| 3 eighths add 1 quarter Students need to read this and recognise that common denominators are required for addition of fractions Preliminary step could be done with fraction wall (rename
1/4 as
2/8) 3 eighths add 2 eighths is 5 eighths
|
|---|
-2 + 5
| minus 2 plus 5
| Negative two add five
Start at –2 on number line and move 5 to the right (ends at 3)
|
|---|
Subtraction
Example
Example
| Common reading
| Alternative reading for meaning
|
|---|
|
5 – 2 | | 5 take away 2
how much more is 5 compared to 2?
what is the difference between 5 and 2?
|
|---|
4 – -1
| 4 minus negative 1
4 subtract negative 1
4 minus minus 1
4 subtract minus 1
| Difference between 4 and negative 1
How much more is 4 compared to -1?
Answer is 5 if both numbers are shown on a number line
|
|---|
Multiplication
Example
Example
| Common reading
| Alternative reading for meaning
|
|---|
|
3 × 5 | 3 times 5
3 multiplied by 5
| Students can read × as 'of'
3 groups of 5, or 3 lots of 5
Repeated addition works for integer values of the multiplier, i.e., the first number.
|
|---|
7 × 1/2
|
| With fractions, students can read × as 'of', so 7 ×
1/2 can be read as 7 lots of
1/2. (repeated addition); show 7 jumps of
1/2 on a number line from 0 to 3
1/2)
Note: using commutativity, same answer as
1/2 of 7
|
|---|
4/7 × 35
| 4 over 7, times 35
| 4 lots of one seventh of 35 (i.e., 4 lots of 5 which is 20)
|
|---|
0.1 × 3.4
| point 1 times 3.4
| one tenth of 34 tenths (1/10 × 34/10) and use fraction multiplication gives
34/100 which can also be written (renamed) as 0.34
|
|---|
2 × –3
| two times minus 3
| start at –3 on the number line, then show twice as many lengths from 0 (–6)
|
|---|
Division
There are two versions of division: partition division and quotition division.
Understanding the language associated with both quotition and partition division can assist students when interpreting division problems.
In either case, there is an optional preliminary step ("rename" or "re-express" a in an alternative way that will make the next step simpler), for example, renaming 1.2 as 12 tenths when dividing by 4.
Further examples are noted below. In particular, the renaming of the first number in quotition division mirrors the process of finding a common denominator (as quotition division is repeated subtraction and subtraction of fractions requires a common denominator).
Partition division
-
a ÷ b can be read as a shared between b people, how much each?
- can be thought of as equal shares, (where b is a positive integer)
- shown as a diagram with the total a being put into b groups (in the manner of dealing cards)
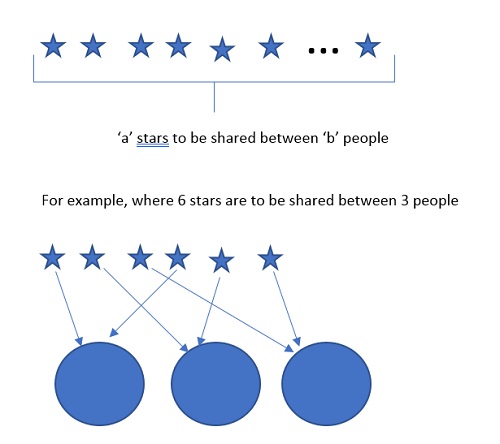An example of partition division
Example
Example
| Common reading
| Alternative reading for meaning
|
|---|
|
2.8 ÷ 7 | 3 times 5
3 multiplied by 52 point 8 divided by 7
| Rename 2.8 as 28 tenths, so the question becomes 28 tenths, shared between 7, how much each?
- Where the answer is 4 tenths each
- Using reasoning of 28 things shared into 7 groups is 4 things each)
|
|---|
0.3 ÷ 10
| | Rename 0.3 as 30 hundredths, so the question becomes 30 hundredths, shared between 10, how much each?
- where the answer is 3 hundredths each
- using reasoning of 30 things shared into 10 groups is 3 things each)
|
|---|
4
1/2 ÷ 3
| 4 and a half divided by 3
| 4 and one half shared between 3, how much each?
- show sharing or "dealing out" of wholes,
- then smaller parts, into 3 groups.
- final shares are 1 and one half, 1.5.
Rename 4 ½ as 9 halves, so the question becomes 9 halves, shared between 3, how much each?
- where the answer is 3 halves each
- using reasoning that 9 things shared into 3 groups is 3 things each
|
|---|
Quotition division
-
a ÷ b can be read as a, how many b?
- can be thought of as repeated subtraction, (where b is smaller than a)
- can be shown as jumps of size b from a back to 0 on a number line
- simplest cases are when the answer is a positive integer (so a is a multiple of b)
Example
Example
| Common reading
| Alternative reading for meaning
|
|---|
|
1.4 ÷ 0.1 | 1 point 4 divided by point 1
| 1 and 4 tenths, how many tenths? - show 14 jumps of 1 tenth on a number line from 1.4 back to 0
14 tenths, how many tenths? - where the answer is 14 without using a number line; note preliminary step
|
|---|
1 1/2 ÷ 1/8
| 1 and a half divided by one eighth
| 1 and a half, how many eighths? - show 12 jumps of 1/8 on a number line from 1 1/2 back to 0
rename 1 1/2 as 12/8; 12 eighths, how many eighths?
- where the answer is 12 without using a number line
|
|---|
Curriculum links for the above examples: VCMNA241, VCMNA243,
VCMNA244,
VCMNA273.