Level 3–4: Multiplicative Thinking
Materials
Instructions
Place 24 counters and 6 lolly-bags in front of student, point to counters and say, “Can you share these out equally so that every lolly-bag has the same amount of counters?”
Note student’s response/strategy.
Place Card A and Card B in front of the student and say, “Without counting can you tell me if there are the same number of dots on each card, or does one card have more than the other? … How do you know?”
If completed relatively easily, say, “Imagine you have 2 lollies but your sister has 3 times as many lollies as you. How many lollies does your sister have?”
Advice Rubric
This task examines the extent to which students understand sharing and the importance of fair shares (equal groups) as a basis for division. It also explores the extent to which students recognise the invariance of the product (in this case, that 3 groups of 4 is the same as 4 groups of 3) and the idea of ‘times as many’.
| Observed response |
Interpretation/Suggested teaching response |
|---|
Little/no response or incomplete (eg, puts one or two counters on each bag but leaves the rest), hesitant/slow to respond to Card task |
May not understand task
- Review idea of sharing and fair shares using appropriate real-world tasks (eg, share out the glue-pots, so that each table has 3 pots)
- Play simple card games that involve dealing out an equal number of cards to each player
|
Distributes counters to bags unequally (eg, makes groups of 6 leaving 2 bags empty or randomly allocates counters to bags). Says there are a different number of dots on each card (either more on Card A because there are more groups or more on Card B because there is more in each group) |
Suggests that task of sharing not understood and/or an inability to discriminate between the number of groups and the number in each group, possibly relying more on perception than reasoning
- Review idea of sharing and fair shares using appropriate real-world activities (eg, sharing resources, playing card games)
- Make and name arrays, rotate and use strategies to demonstrate equivalence (eg, 3 rows of 5 is double 5, and 5 more, 15 … 5 rows of 3 is double, double 3 (12) and 3 more, 15)
- Play Multiplication Toss (pdf - 48.7kb) to practice making and naming regions and recognising the commutativity of multiplication
|
Arrives at correct solution (4 counters/bag) by trial and error. For Card task may say that there are a different number of dots initially but either counts or uses known facts to correct response. Adds (2+2+2) to find number of lollies. |
May not understood value of systematically sharing to ensure equal outcome, may be uncertain about equivalence of different representations
- Review systematic sharing strategies and importance of fair-shares (eg, explore collections such as 24, find and name the different ways in which it can be shared equally, ie 2 twelves, 3 eights, 4 sixes, 6 fours, 8 threes, 12 twos, discuss how we can be sure that we have found them all)
- Introduce array-based strategies for multiplication facts, eg, for 2s facts (2 ones, 2 twos, 2 threes, …) think doubles, for 3s facts (3 ones, 3 twos, 3 threes, …) think double the group and one more group etc (see above)
- Play Multo (Maths300, Curriculum Corporation) to reinforce the idea that products may be represented in different ways (eg, 18 is 6 threes, 3 sixes, 9 twos, or 2 nines)
|
Shares systematically or uses known fact (24 divided by 6 is 4), to arrive at 4/bag. Recognises cards have the same number of dots and determines the number of lollies multiplicatively (3 twos) |
Suggests a sound understanding of initial ideas for multiplication
- Consolidate mental strategies for multiplication to 100 and beyond (eg, 9 twenty-threes, think: less than 10 twenty-threes (230), one group less, 207)
- Introduce symbolic recording for basic facts and discuss informal strategies for multiplying larger numbers by single digit numbers
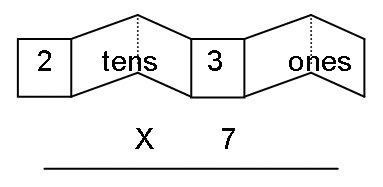
- Consider introducing the area idea for multiplication by using MAB (Base 10 blocks) to explore 1 digit by 2 digit multiplication (eg, represent 7 by 23 as 7 rows of 2 tens and 3 ones), discuss convenience of this as opposed to 7 rows of 23 ones
- Use Number Expanders to support recording and working with place-value parts (eg, 7 by 3 ones … 7 by 2 tens)
|