Level 3: Multiplicative Thinking
Materials
Instructions
Show Card 1 and say, “This is a different way of showing what we get when we add up these numbers.” Indicate 3, 2 and 4, then point to 9 and say, “Do you agree that if we added these numbers up this will be the answer?” Indicate 9. If the student agrees and appears to understand the task, proceed to Card 2.
Place Card 2 in front of the student and ask, “The answer is missing from this card. Can you add up the numbers to find the answer please?… How did you work that out?” Note student’s response then remove the card. If answered relatively easily proceed to Card 3, otherwise stop at this point.
Place Card 3 in front of the student and say: “This time the answer is there (point to 24), but one of the numbers is missing. Can you work out what number is missing please? … How did you work that out?”
If this was done relatively easily, remove Card 3 and place Card 4 in front of the student. Ask, “What do you think needs to be done here? … Can you do that for me please? … Can you tell me how you did that?” If student hesitates or find this difficult, stop and try to find out why.
If this was done easily, proceed to Card 5 and repeat the questions.
3.2 Advice rubric
This task assumes some facility with addition and subtraction facts to 20. It examines the extent to which students have access to efficient mental strategies to add and subtract 1 and 2 digit numbers to 30 and beyond, which is an important pre-requisite for developing efficient mental strategies for the multiplication facts to 100.
| Observed response |
Interpretation/Suggested teaching response |
|---|
Little/no response to Card 1, counts to 9 by ones or uses fingers |
May not understand task and/or ‘trust the count’ for single-digit numbers shown, may not be able to keep track of the count
- Use subitisation cards to check part-part-whole understanding for numbers to 5 and the extent to which students can recognise and work with numerals to 5 without having to model or count by ones (trusting the count)
- Build number fact knowledge (and trusting the count) to 10 using subitisation cards and ten-frames (ie, recognise 7 is 5 and 2 more, 3 and 4, 1 more than 6, and so on).
- Use two large dot dice and/or ten-frame cards to model counting on (ie, cover number that is known and count on by ones), extend to counting on by 1, 2 or 3 mentally
- Use 2-row bead frames and ten frames to build doubles knowledge to 20
|
Experiences difficulty with Card 2 (eg, takes a long time, uses fingers, taps, nods), and/or indicates that they counted by ones |
Suggests little or no access to mental strategies beyond count on by 1, 2, or 3 from larger
- If Students can subitise numbers up to 5, continue building part-part-whole knowledge of numbers to 10 as above, using subitisation cards and ten-frames eg 17 is 1 ten and 7 ones
- Develop more efficient addition strategies for number facts to 20: count-on, count-on-from-larger, doubles and near doubles (eg, for 8 and 9, double 8 is 16 and one more is 17), make-to-ten (eg, for 6 and 8, simultaneously recognise 8 is 2 less than 10 and 6 is 2 and 4, so 8. 10. 14)
- Use ‘thinking strings’ to model addition of three or more digits (eg, for 8 and 5 and 7, record: “8, 10, 13, 20”)
|
Able to find the sum (23) for Card 2 and the missing number (12 ) for Card 3 but experiences difficulty with Card 4 |
Suggests a knowledge of addition facts to 20 and/or access to relatively efficient mental strategies for adding and subtracting single digit numbers.
- Extend doubling/near doubling strategies to 2-digit numbers emphasising the count of tens (eg, for double 24, think: double 2 tens is 4 tens , double 4 ones is 8 ones, so 48)
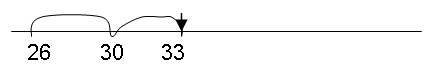
- Use open number lines and thinking strings to extend the ‘make-to-ten’ strategy, eg, for 26 and 7 and counting in place-value parts, eg, for 36 and 47, start at 47, count on 3 tens, 87, and 6 more, 87, 90, 93
- Use similar cards to model and develop ‘inspection’ strategies for adding and subtracting 3 two-digit numbers (eg, for Card 4, 3 tens and 7 tens is 10 tens and 5 more tens is 15 tens, 8 and 2 gives 1 more en 16 tens and 4 more, 164)
- Introduce/consolidate column addition for 2 or more addends and trading strategies to support written recording for subtraction
- Consider introducing array-based strategies for multiplication facts, eg, for 2s facts (2 ones, 2 twos, 2 threes, …) think doubles, for 3s facts (3 ones, 3 twos, 3 threes, …) think double the group and one more group etc (See Developing the Big Ideas in Number (PDF - 125Kb) (pdf - 125.23kb))
|
Completes all cards reasonably efficiently |
Indicates sound knowledge of addition and subtraction facts and access to flexible mental strategies for adding and subtracting 2-digit numbers.
- Consolidate written recording for addition and subtraction with regrouping and trading
- Extend multiplication strategies to 100 and beyond (eg, 9 twenty-threes, think: less than 10 twenty-threes (230), one group less, 207)
|